© 2008 – 2012 Gwen Dewar, all rights reserved
What do babies know about numbers?
Back in the 20th century, people assumed that infants lacked “number sense.”
But today’s cognitive scientists have overturned the old ideas.
Experimental research reveals a fascinating new world of baby cognition, one in which babies can
- recognize the approximate difference between two numbers
- keep precise track of small numbers, and
- do simple subtraction and addition problems.
Moreover, when babies perform these feats they activate the same parts of the brain that are associated with mathematical thinking in adults.
Here I review recent discoveries. I begin with what babies know about numbers—big and small—and end with a discussion of baby “arithmetic” and the brain.
What babies know about “numerosity”
Psychologists define “numerosity” as the number of things in a set.
Although we can get a precise measure of numerosity by counting, it’s possible to appreciate numerosity in a more approximate way.
For instance, if I ask you to glance in a crowded elevator and estimate how many people are there, you can probably make a pretty good guess.
Glance inside two elevators, and you’ll also be pretty good at guessing which one has more people in it.
All without counting.
It turns out that adult humans aren’t the only creatures who can pull this off. A variety of non-verbal creatures–including monkeys, rats, fish, and human infants–can detect the approximate difference in magnitude between two sets (Dehaene 1999; Piffer et al 2012).
Show them two displays—one featuring 8 items and the other only 4 items—and they will respond differently depending on what they see.
And the difference is observable in the brain.
When 3-month old infants were shown a series of displays depicting different quantities of objects, the electrical activity of their brains changed in response (Izard et al 2008).
For example, babies were presented with a continuous stream of images, each depicting 4 objects. From image to image, the objects were arranged differently. But the total number of objects remained the same (see the first four slides in the image below).
Infants subjected to this program showed signs of boredom. But their brain activity (as measured by event-related potentials, or ERPs) would perk up if they were next presented with an image showing 8 objects (see the fifth, “test” slide in the image above).
What’s more, the part of the brain that was activated—the parietoprefrontal network—was the same region that lights up when adults process information about numbers (Izard et al 2008).
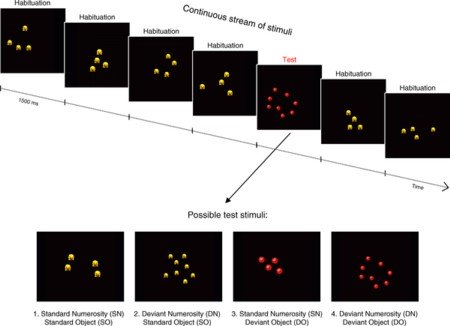
A strong case for “baby number sense”
Were the babies really responding to the change in numerosity? It certainly seems so.
Babies reacted similarly when presented with pairs of small numbers (2 vs 3) and distant, large numbers (4 vs 12).
And researchers controlled for several, non-numerical variables that could have influenced babies—like the total surface area of the objects, the average area devoted to each object, the total luminance of each image, and the total area occupied by each group of objects.
So babies weren’t simply attending to the continuous extent of “stuff” in each image.
In fact, other experiments suggest that babies pay more attention to change in number than they do to changes in continuous extent or surface area (Cordes and Brannon 2008).
Nor is it all “in the eye.” If babies’ feats of numerical discrimination were confined to visible objects, we might suspect that the ability is domain specific—a specialization of the visual system rather than a more general conceptual ability.
But babies aren’t one-trick ponies. In addition to distinguishing visual arrays, babies can also detect differences in the number of sounds and in the number of actions (Lipton and Spelke 2003; Wood and Spelke 2005).
Babies can even understand ordinality–the idea that numbers can be arranged in order of magnitude. When 11-month old babies were presented with sequences of numerosities, they could discriminate between sequences that increased and sequences that decreased (Brannon 2002).
Of course, this doesn’t mean that babies can distinguish any two numbers. There are limits. For instance:
- Six-month old babies have a hard time distinguishing two quantities if the ratio between them is less than 2:1. In other words, they can handle 8 vs. 16, but fail to distinguish 8 vs. 12 (Xu and Arriaga 2007; Lipton and Spelke 2003).
- Nine- and 10-month olds can make finer distinctions (8 vs. 12). But even these older babies don’t seem to discriminate between 8 and 10 (Xu and Arriaga 2004; Lipton and Spelke 2003).
- Babies don’t distinguish between increasing and decreasing sequences of numbers until the approach the end of their first year. When Elizabeth Brannon tested 9-month old babies, they failed the test (Brannon 2002).
- Babies’ have been stumped by experiments asking them to distinguish a very small number from a larger one (e.g., 2 vs 4 or 2 vs 8). Kristy Van Marle (2013) thinks that’s because babies rely on different mechanisms to make judgments: A precise tracking mechanism for numbers smaller than 4 and an approximate system for judging rough magnitude. Babies don’t know yet how to integrate the two data sets.
Nevertheless, the results are pretty impressive. When babies look at a set of objects, they don’t just see a “bunch.” Babies have an approximate sense of number.
Do babies act on what they know?
It seems that babies appreciate differences in magnitude. But can babies put this knowledge to any use? Clever experiments suggest that they can.
In one study, 14-month old babies watched experimenters place toys in a box. Then experimenters asked the babies “What’s in the box?” and let them search.
If a baby had seen only one toy placed in the box, he tended to stop searching after he retrieved that ball. But if he’d seen two balls go in, he’d search longer. Using this method, researchers reported that babies could keep track of up to three items (Feigenson and Carey 2003).
In similar experiments, 21-month old babies watched while an experimenter placed balls in a box one at a time (Langer et al 2003). Then they were given these instructions:
“One at a time, with one hand, take all the balls out of the box…”
If babies had seen more than one ball placed in the box, they reached into the box more than once.
Experimenters also ran a trial in which babies saw the experimenter place two balls in the box, and then pull one ball back out. After watching these trials, babies who were asked to “take all the balls out of the box” reached into the box only once.
Baby arithmetic?
These “balls in the box” experiments suggest that babies can perform other feats–namely, very basic calculations.
Placing two balls in a box–one after the other–amounts to an exercise in addition (1+1). Similarly, removing one of two balls from a box constitutes subtraction.
So babies who watch these scenarios—and form the correct inferences—are perhaps performing primitive math.
Or are they?
Some researchers have argued that babies don’t solve these problems by doing addition or subtraction. Instead, they are relying on an automatic system for tracking objects.
This system (which has been studied extensively in adults) appears to track individual objects over time by opening a temporary “file” for each one. The brain updates these files as objects move or change (Kahneman et al 1992).
The system is limited by memory constraints—the average person being able to track a maximum of 4 objects at once. But it’s an effective system for coping with very small numbers.
According to the object file theory, babies detect the sudden absence of an object because there is no longer a match between the set of objects and the set of opened object “files.”
Similarly, the brain notices the unexpected appearance of a new object for which there is no file.
It’s not really arithmetic, then. More like a perception and memory task.
But even if babies use object files, we can’t rule out the possibility that babies really do understand something about addition and subtraction.
To resolve the question, we need to test babies’ knowledge of arithmetic without involving their object tracking systems.
And that’s exactly what Koleen McCrink and Karen Wynn set out to do.
What babies know about addition and subtraction
Cognitive scientists McCrink and Wynn suspected that babies really could perform numerical computations–if only in a rough, approximate way.
To test their idea, they presented 9-month old babies with operations involving large number, numbers too large for the object tracking system to handle (McCrink and Wynn 2005).
During the experiment, researchers showed babies several animated films depicting a group of moving rectangles.
In films presenting the addition scenario, 5 rectangles moved around the screen and then hid behind an occluder. Next, 5 more rectangles followed. The movies ended in one of two ways:
- the occluder moves off to reveal 10 rectangles (the correct outcome), or
- the occluder moves off to reveal 5 rectangles (the incorrect outcome).
The subtraction scenario was similar, except that it began with 10 rectangles that hid behind an occluder. Subsequently, 5 rectangles slide back “out” and traveled—one by one—off the screen. The movies ended with either
- the occluder moving off to reveal 5 rectangles (the correct outcome), or
- the occluder moving off to reveal 10 rectangles (the incorrect outcome).
In both scenarios, babies stared longer at the screen when it displayed the incorrect outcome.
The implications? Babies didn’t expect the wrong answers.
They weren’t looking longer because they preferred a certain number of rectangles. The incorrect outcomes were associated with different numbers (5 for the addition scenario, 10 for the subtraction scenario).
So it seems that the babies understood two basic points—that addition makes a set more numerous, and subtraction makes it less numerous.
Wynn and McCrink concluded that these babies really do have procedures for numerical calculation, procedures that don’t depend on the automatic object-tracking system.
So how do babies do it?
It appears that babies–like adults and like many non-human animals–can track very small sets of objects automatically. They also have the capacity to compare large sets in an imprecise way.
The two feats are distinct and get processed in different parts of the brain. A study measuring brain activity in 7-month infants reports distinct neural signatures associated with each task (Hyde and Spelke 2011).
And it appears there are specialized neurons for keeping track of approximate quantities greater than 3.
Experiments on monkeys have identified “accumulator neurons,” brain cells that are activated by visual displays of objects. The more objects on display, the more these special neurons respond (Roitman et al 2007).
The monkey accumulator neurons were found in the same part of the brain that is associated with number-processing in humans. This region–the lateral surface of the parietal cortex–has also been linked with the ability to locate objects in space and time.
So when your baby looks at a large array of objects, it seems likely that specialized accumulator neurons are firing off in her parietal cortex. The neurons respond in a graded manner, and the extent of their activity supplies her brain with an approximate representation of number.
And the result? A baby that knows the difference between 4 and 8 cookies. Even before she knows how to ask for them by name.
More reading
My article about early childhood math lessons talks about how to use what babies know about numbers as a springboard for introducing toddlers to mathematics.
And for more information about the minds of babies, check out this collection of Parenting Science articles.
References: What babies know about numbers
Brannon EM. 2002. The development of ordinal numerical knowledge in infancy. Cognition 83(3):223-40.
Brannon EM, Abbott S, and Lutz DJ. 2004. Number bias for the discrimination of large visual sets in infancy. Cognition. 93(2):B59-68.
Cordes S and Brannon EM. 2008. The difficulties of representing continuous extent in infancy: using number is just easier. Child Development 79(2):476-89.
Feigensen L, Carey S, and Hauser MD. 2002. The representations underlying infants’ choice of more: object files versus analog magnitudes. Psychological Science 13: 150-156.
Hyde DC and Spelke ES. 2011. Neural signatures of number processing in human infants: evidence for two core systems underlying numerical cognition. Dev Sci. 14(2):360-71.
Izard V, Dehaene-Lamberz G, and Dehaene S. 2008. Distinct cerebral pathways for object identity and number in human infants. PLoS Biol 6(2):e11.
Kahneman D, Treisman A, and Gibbs. 1992. The reviewing of object files: Object-specific integration of information. Cognitive Psychology 24: 175-219.
Langer J, Gillette P, and Arriaga RI. 2003. Toddlers cognition of adding and subtracting objects in action and perception. Cognitive Development 18: 233-246.
Leon MI and Gallistel R. 1998. Self-stimulating rats combine subjective reward magnitude and subjective reward rate multiplicatively. Journal of Experimental Psychology: Animal Behavior Processes 24(3):265-77.
Lipton JS and Spelke ES. 2003. Origins of number sense: Large number discrimination in human infants. Psychological Science 14(5): 396-401.
McCrink K and Wynn K. 2004. Large-number addition and subtraction by 9-month-old infants. Psychol Sci. 15(11):776-81.
Piffer L, Agrillo C, and Hyde DC. 2012. Small and large number discrimination in guppies. Anim Cogn. 2012 Mar;15(2):215-21.
Roitman, J., Brannon. E.M. & Platt, M.L. (2007). Monotonic Coding of Numerosity in Macaque. PLoS Biology, 5(8).
Vanmarle K. 2013. Infants use different mechanisms to make small and large number ordinal judgments. J Exp Child Psychol. 114(1):102-10.
Xu F and Arriaga RI. 2007. Number discrimination in 10-month-old infants. British Journal of Developmental Psychology 25: 103-108.
Xu F, Spelke ES and Goddard S. 2005. Number sense in human infants. Dev Sci. 8(1):88-101.
content of “What babies know about numbers” last modified 12/12
Image displaying example of slide sequences shown to infants © 2008 Izard V, Dehaene-Lamberz G, and Dehaene S. Distinct cerebral pathways for object identity and number in human infants. PLoS Biol 6(2):e11.